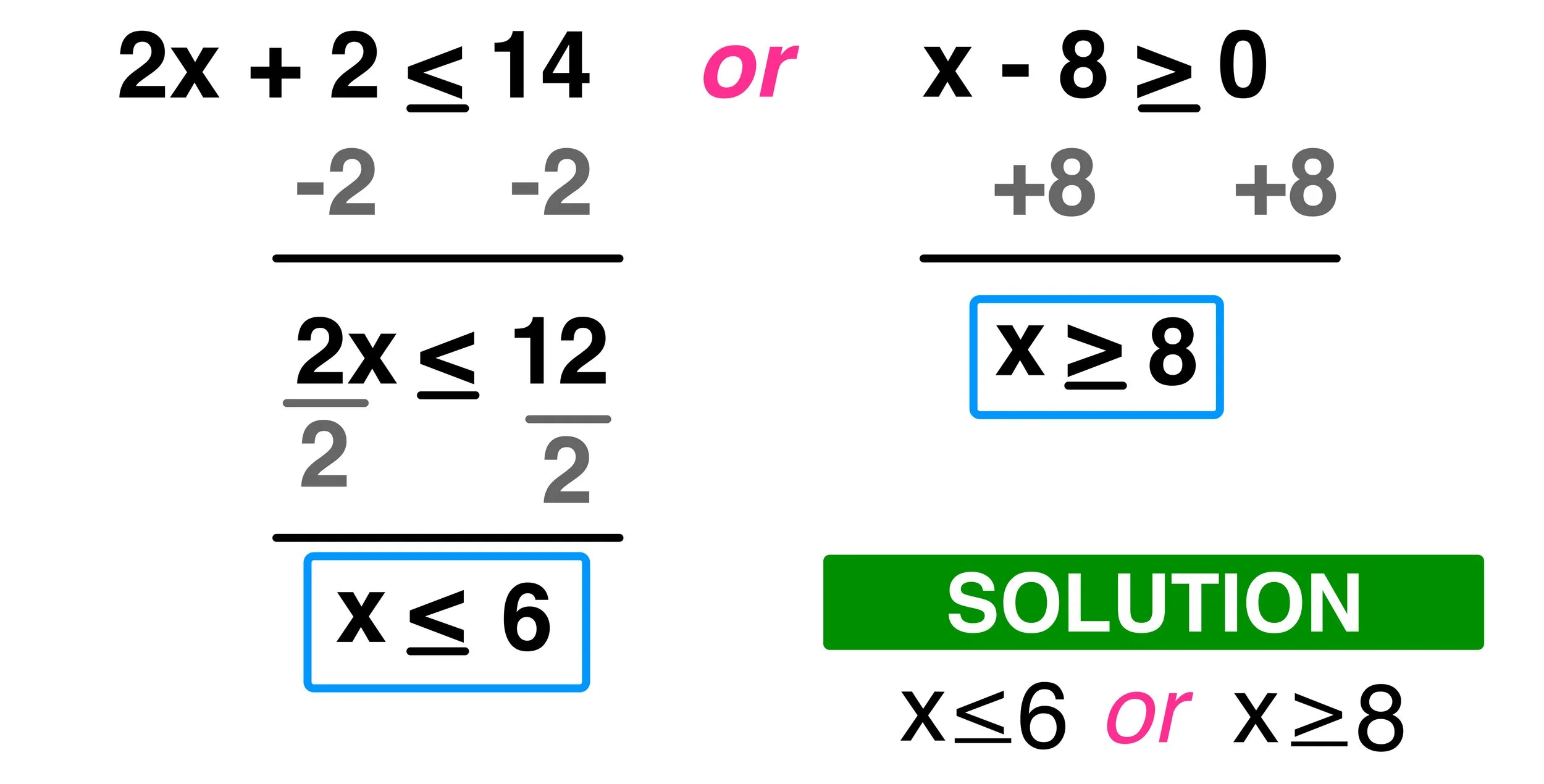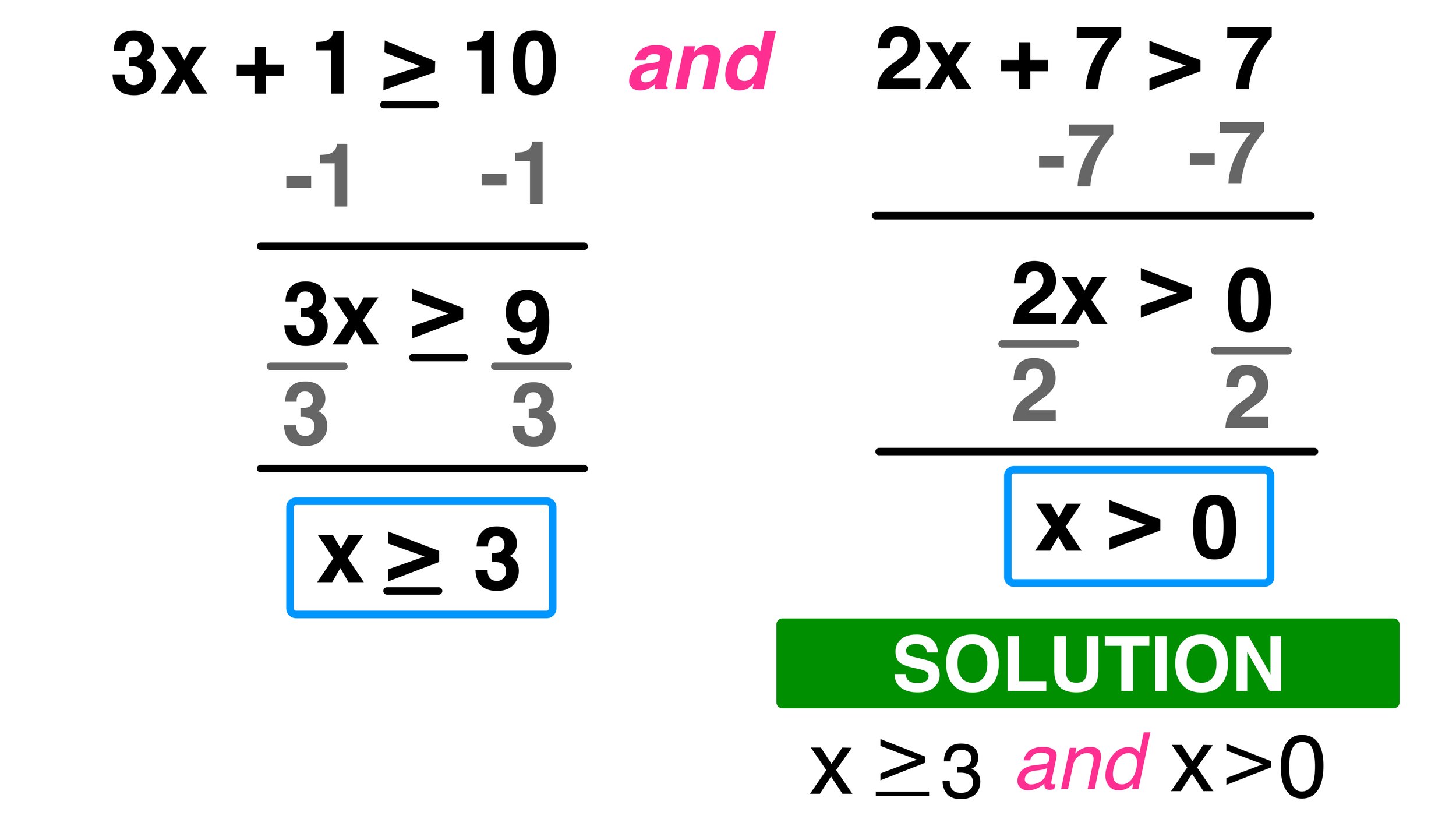How to Solve Compound Inequalities in 3 Easy Steps
Being able to create, analyze, and solve a compound inequality using a compound inequality graph is an extremely important and helpful math skill that can be applied to many math concepts commonly found in pre-algebra, Algebra I, Algebra II, and even Pre-Calculus and Calculus. While many students may be intimidated by the concept of a compound inequality when they see unusual looking graphs containing circles and arrows, but working with compound inequalities is actually quite simple and straightforward.
The following free How to Solve Compound Inequalities step-by-step lesson guide will teach you how to create, analyze, and understand compound inequalities using an easy and effective three-step method that can be applied to any math problem involving a compound inequality or a compound inequality graph. As a student, if you can follow the three steps described in this lesson guide, you will be able to easily and correctly solve math problems involving compound inequalities.
Are you ready to get started? Before you learn about creating and reading compound inequalities, let’s review a few important vocabulary words and definitions related to inequalities.
What is an inequality?
Before we explore compound inequalities, we need to recap the exact definition of an inequality how they compare to equations.
What is an equation?
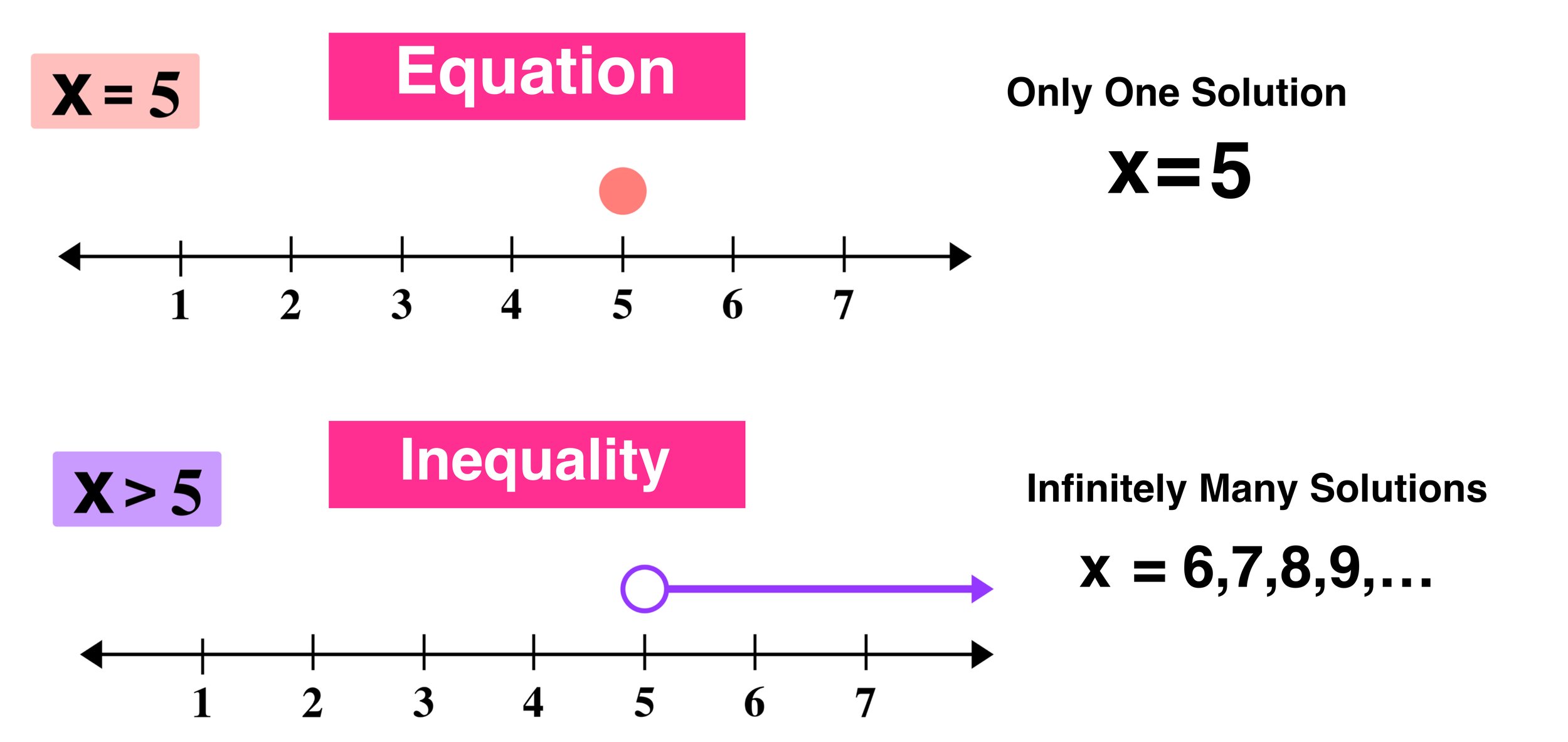
Definition: In math, an equation is a statement that shows that two mathematical expressions are equal to each other using an “=” sign.
For example, x=5 is an equation where the variable and x are equal to a value of 5 (and no other value).
It is important to note that equations are limited to only one possible solution, so, in this case, 5 is the only possible value that x can be equal to, and any other value would not apply.
x=5
The only solution: 5
What is an inequality?
Definition: In math, an inequality is a relationship between two expressions or values makes a non-equal comparison.
For example, x>5 is an inequality that means “x is greater than 5,” where, unlike an equation that has only one solution, x can have infinitely many solutions, namely any value that is greater than 5.
We can visualize the simple inequality x>5 on the number line below as follows:
In comparison to equations, inequalities are not limited to only one possible solution. In fact, inequalities have infinitely many solutions.
In this case, solutions to the inequality x>5 are any value that is greater than five (not including five).
x>5
Examples of solutions: 6, 7, 10, 105, 2,500 (all of these values satisfy the inequality because they are greater than 5)
Examples of non-solutions: 5, 4, 0, -17, -1,001 (none of these values satisfy the inequality because they are not greater than 5)
What is the difference between an equation and an inequality?
Before we move onto exploring inequalities and compound inequalities, it’s important that you understand the key difference between an equation and an inequality.
In essence, the key difference is between an equation and an inequality is:
an equation has one and only one solution
an inequality has multiple solutions
Graphing Inequalities on the number line
Now that you understand the difference between and equation and an inequality, you are ready to learn how solve compound inequalities and read compound inequality graphs.
But first, let’s quickly recap how to graph simple inequalities on the number line.
There are four types of inequality symbols:
>: greater than
<: less than
≥: greater than or equal to
≤: less than or equal to
It is important to understand the differences between these symbols, namely the significance of the line underneath a greater than or less than symbol and how it relates to the solution of an inequality and its graph on the number line.
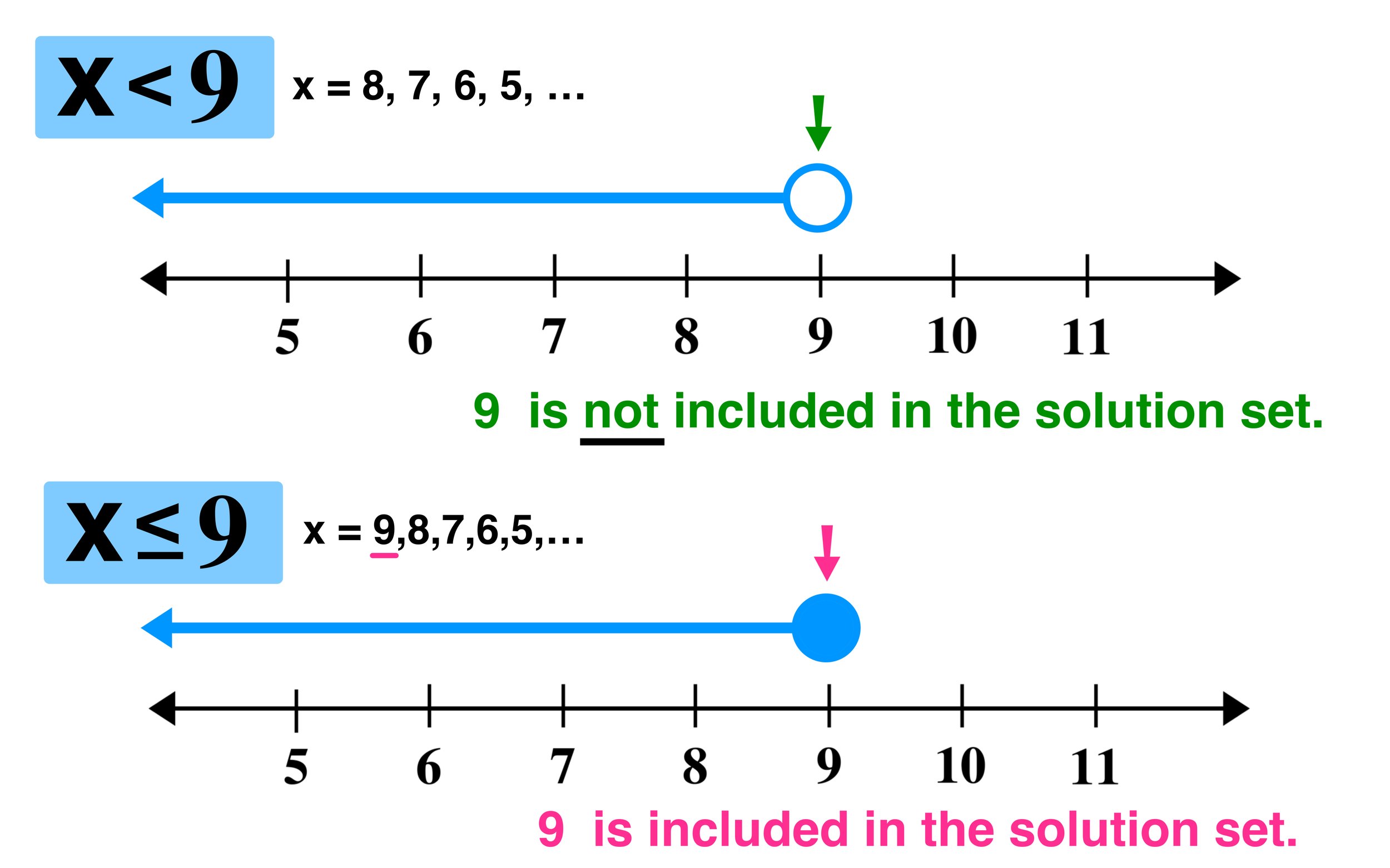
For example, consider the following inequalities: x < 9 and x ≤ 9
The first inequality, x<9, has a solution of any value that is less than 9, but not including 9 (since 9 is not less than 9).
The second inequality x ≤ 9, has a solution of any value that is less than 9 AND the value 9 itself (since 9 is greater than or equal to 9).
On the number line, the difference between these two types of inequalities is denoted by using an open or closed (filled-in circle). The open circle means that the corresponding value is not included in the solution set, while the closed circle means that the corresponding value is included in the solution set.
Before moving forward, make sure that you fully understand the difference between the graphs of a < or > inequality and a ≥ or ≤ inequality. Understanding the difference in terms of the solution and the graph is crucial for being able to create compound inequality graphs and solving compound inequalities.
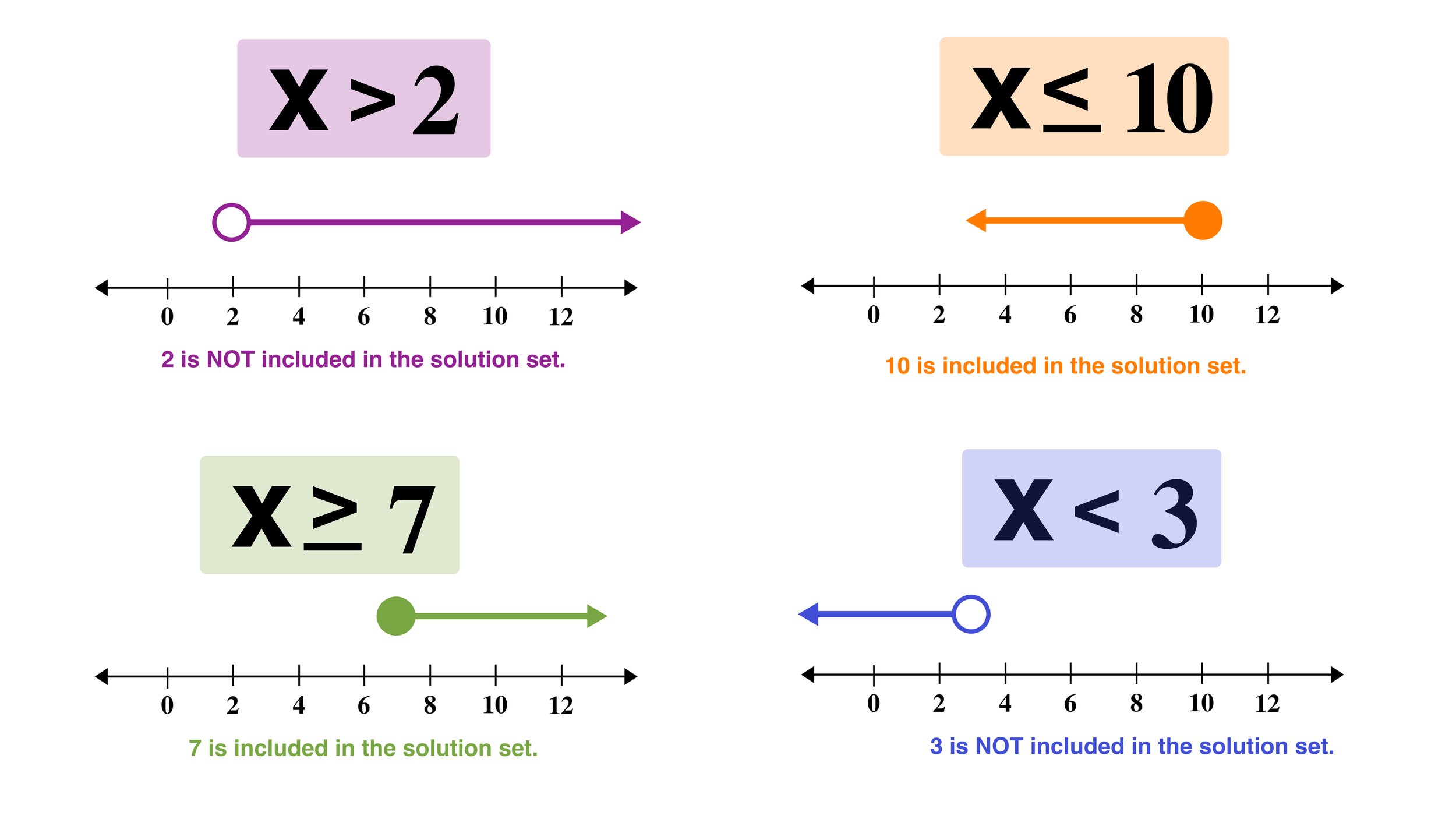
For your reference, here are a few more examples of simple inequality graphs:
Again, an open circle means that the corresponding number line value is NOT included in the solution set. A filled-in circle means that it is included in the solution set.
What is a compound inequality? How to solve compound inequalities?
In the previous section of this guide, we reviewed how to graph simple inequalities on a number line and how these graphs represent the solution to one single inequality.
Definition: A compound inequality (sometimes referred to as a combined inequality) is two simple inequalities joined together.
Is it really that simple? Yes! A compound inequality is just two simple inequalities combined together and a compound inequality graph is just two simple inequalities graphed on the same number line.
There are two types of compound inequalities: or and and.
Definition: An or compound inequality uses the word “or” to combine two inequalities.
Definition: An and compound inequality uses the word “and” to combine two inequalities.
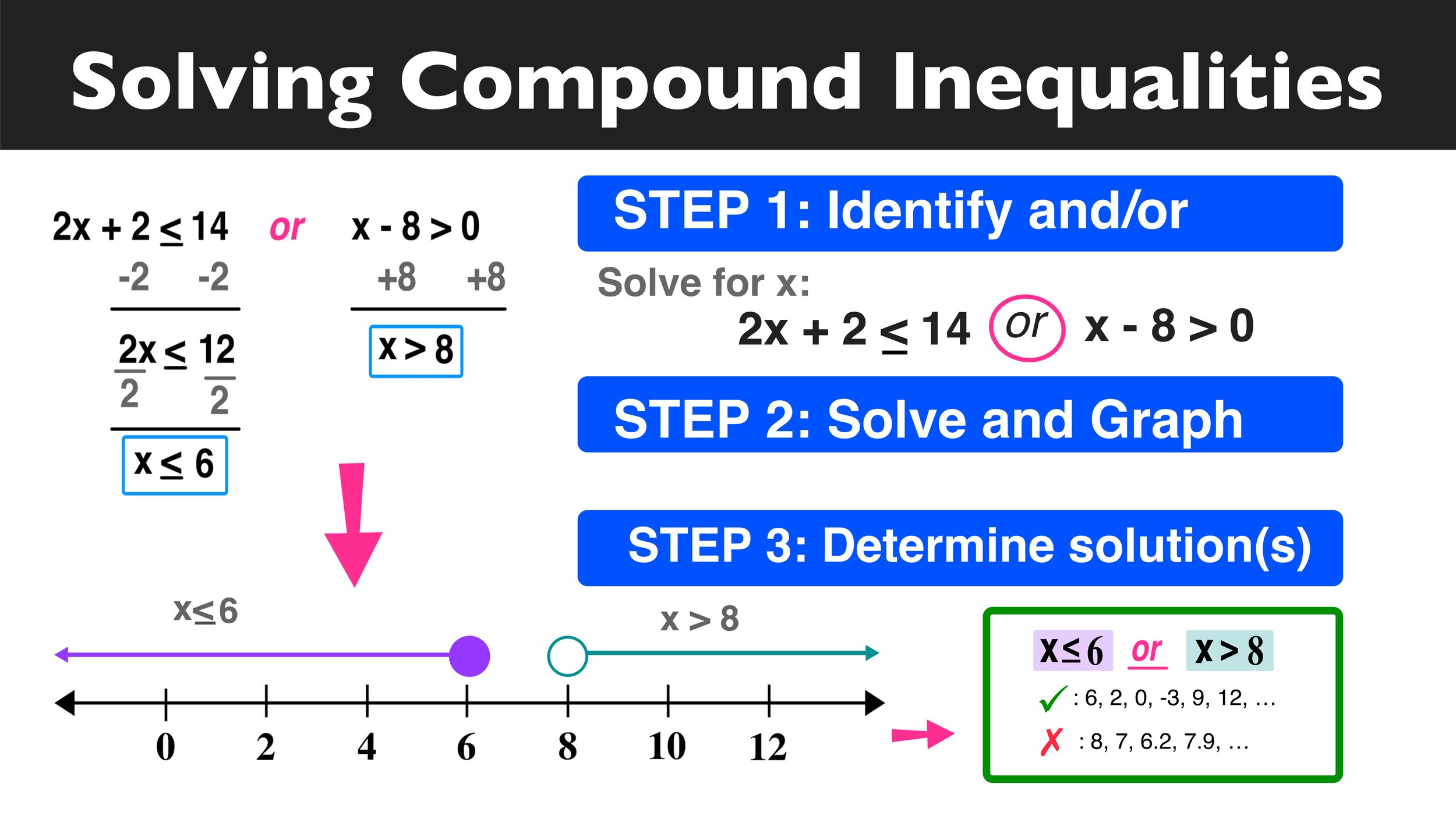
To understand the difference between or and and inequalities, let take a look at a few examples apply the following 3-step process:
Step #1: Identify if the solving compound inequalities problem is or or and
Step #2: Graph both inequalities on the number line.
Step #3: Analyze and determine the solution set.
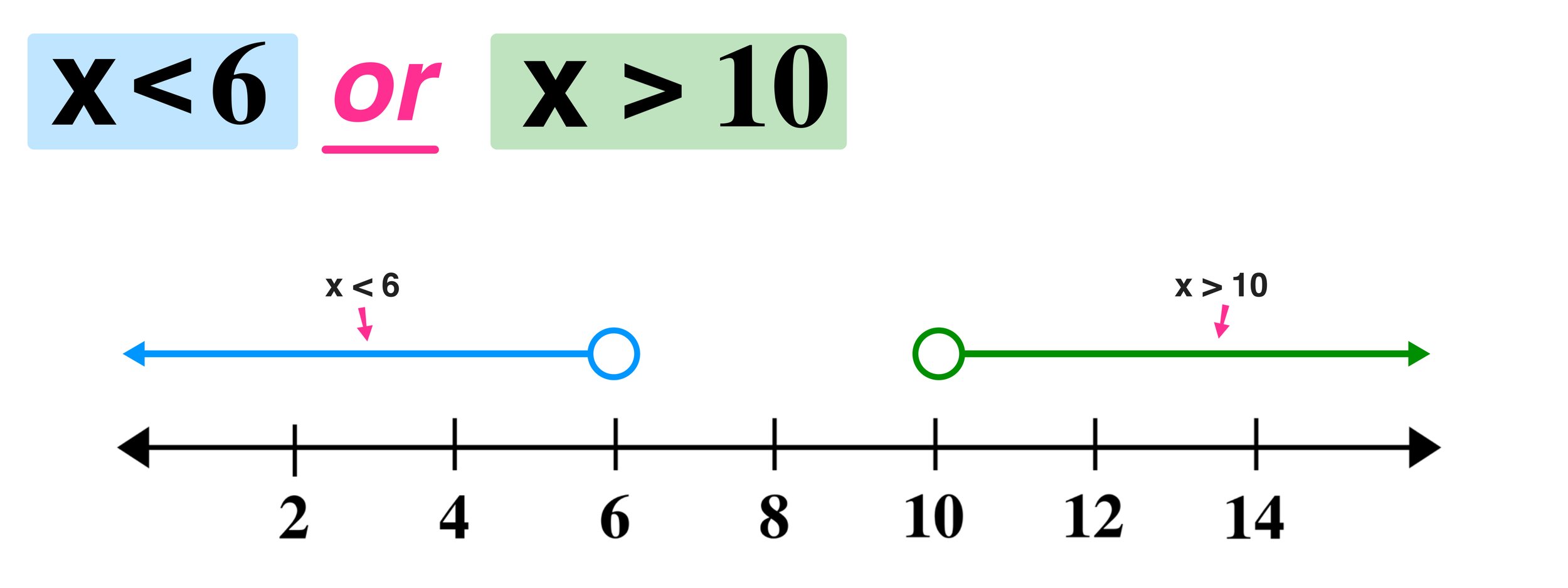
How to Solve Compound Inequality Graphs Example #1: or
Example #1: Graph the compound inequality x<6 or x>10
Step #1: Identify if the solving compound inequalities problem is or or and
Step one is simple since every example will include the word or or and. In this first example, the word or is used, so make a note of that and move forward.
Step #2: Graph both inequalities on the number line.
Next, graph both simple inequalities x<6 and x>10 on the number line to create the following compound inequality graph.
Step #3: Analyze and determine the solution set.
Now that you have your graph, you can determine the solution set to the compound inequality and give examples of values that would work as solutions as well as examples of non-solutions.
For example, the values 4 and 14 are both solutions to this compound inequality, by the number 8 is not a solution.
Additionally, the values 6 and 10 are not solutions since they are included in the solution set since the circles are open.
The shaded area in the graph below represents the solution areas of the compound inequality graph.
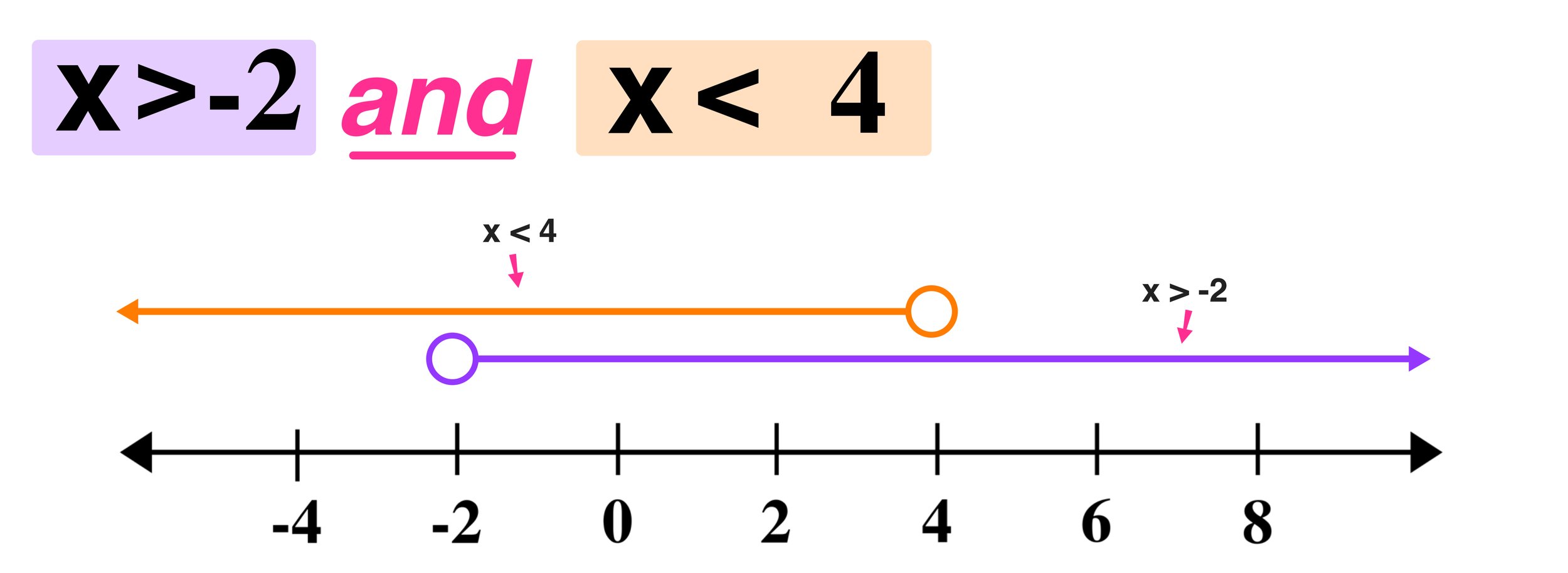
How to Solve Compound Inequality Graphs Example #2: and
Example #2: Graph the compound inequality x>-2 and x < 4
Step #1: Identify if the solving compound inequalities problem is or or and
Notice that this example uses the word and, so keep this in mind as it will affect how you analyze the solution to the compound inequality in step 3.
Step #2: Graph both inequalities on the number line.
Next, graph both simple inequalities x>-2 and x<4 on the number line to create the following compound inequality graph.
Step #3: Analyze and determine the solution set.
This compound inequality has solutions for values that are both greater than -2 and less than 4.
So, for example:
0 is a solution because it satisfies both x>-2 and x<4.
-4 is not a solution because it is only a solution for x<4 (a value must satisfy both inequalities in order to be a solution to this compound inequality)
8 is also not a solution since it does not satisfy both inequalities.
Note that this compound inequality can also be expressed as -2 < x < 4, which means that x is greater than -2 and less 4 (or that x is between -2 and positive 4).
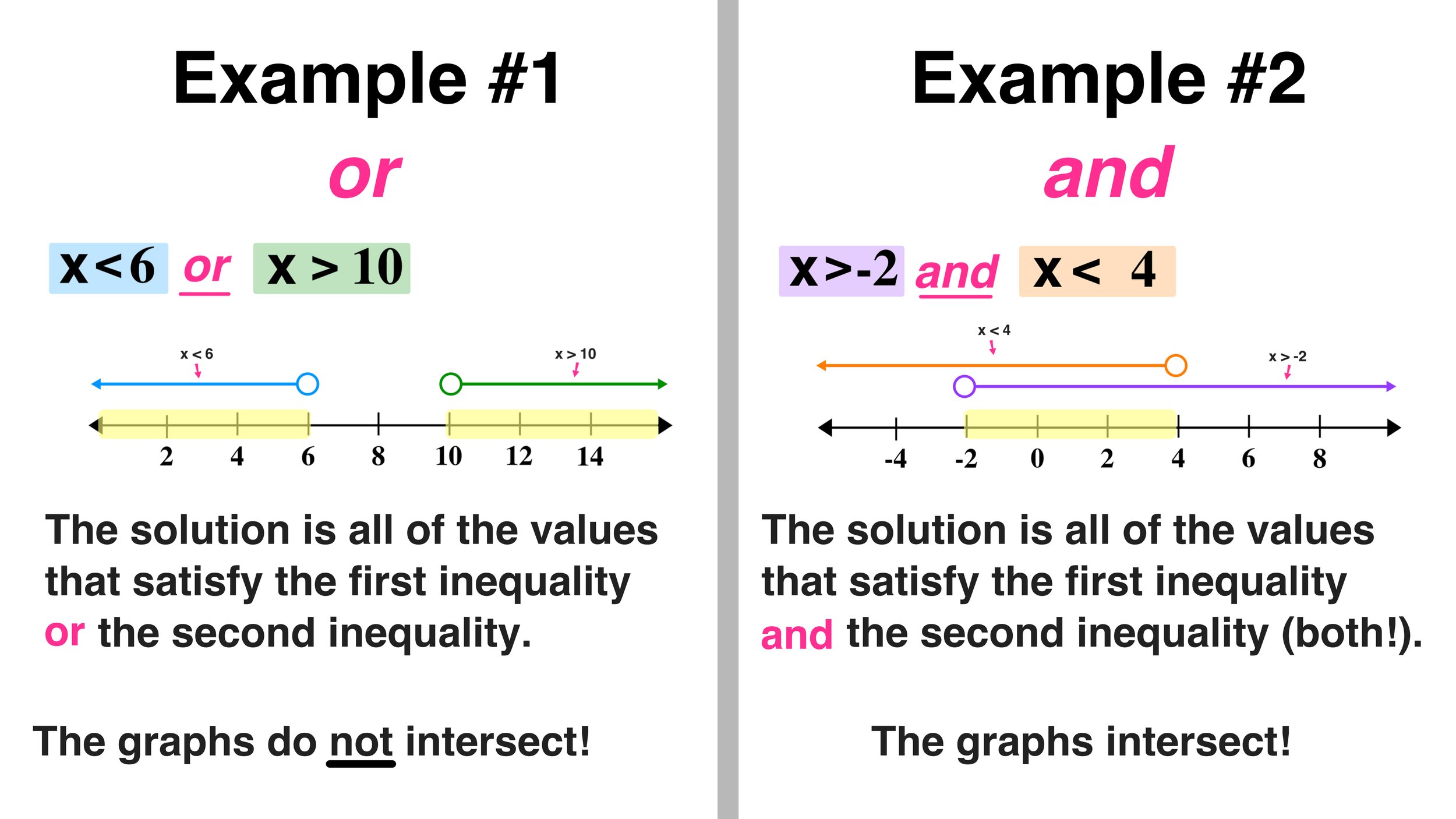
How to Solve Compound Inequality Graphs: or vs. and
Based on the last two examples, did you notice the difference between or and and compound inequalities.
Let’s compare the two graphs again:
The key difference here is that:
The solution to or is examples are values that satisfy the first inequality or the second inequality. Notice that the compound inequality graphs never intersect (overlap).
The solution to and examples are values that satisfy both the first inequality and the second inequality. Notice that the compound inequality graphs do indeed intersect (overlap).
Now, let’s take a look at three more examples that will more closely resemble the types of compound inequality problems you will see on tests and exams:
Solving Compound Inequalities Example #3:
Solve for x: 2x+2 ≤ 14 or x-8 ≥ 0
Don’t panic if this question looks tricky. You will still follow the exact same 3-step process used in examples 1 and 2, but you just have to do a little bit of algebra first.
In this case, before you use the three-step method, solve each inequality to isolate x as follows:
Now you are ready to apply the three-step method for x≤6 or x ≥ 8
Step #1: Identify if the solving compound inequalities problem is or or and
You already know that this is an or compound inequality, so the graph will not have any overlap and any possible solutions only have to satisfy one of the two inequalities (not both).
Step #2: Graph both inequalities on the number line.
Notice that greater than or equal to and less than or equal to symbols are used in this example, so your circles will be filled in as follows:
Step #3: Analyze and determine the solution set.
Again, solving compound inequalities like this require you to determine the solution set, which we already figured out was x≤6 or x ≥ 8.
So, for example, here are a few examples of solutions and non-solutions:
10 is a solution because it satisfies one of the inequalities (x ≥ 8)
6 is a solution because it satisfied ones of the inequalities (x≤6)
7 is not a solution because it does not satisfy either inequality. This also applies to non-solutions such as 6.1 and 7.75.
Solving Compound Inequalities Example #4:
Solve for x: 3x+1 ≥ 10 and 2x+7 > 7
Just like the previous example, use your algebra skills to solve each inequality and isolate x as follows:
Are you getting more comfortable with solving compound inequalities? Notice that the solution to this compound inequality is all values that satisfy x≥3 and x>0.
Now let’s go ahead and follow our three-step method:
Step #1: Identify if the solving compound inequalities problem is or or and
Since this is an and compound inequality, we know that all solutions must satisfy both x≥3 and x>0
Step #2: Graph both inequalities on the number line.
Step #3: Analyze and determine the solution set.
Notice the intersection (or overlap area) of your compound inequality graph:
You can see that all of the solutions to this compound inequality will be in the region that satisfies x≥3 only, so you can simplify your final answer as:
Solution: x≥3
Additionally, here are a few examples of solutions and non-solutions:
5 is a solution because it satisfies both inequalities x x≥3 and x>0
3 is a solution because it satisfies both inequalities x x≥3 and x>0
2 is not a solution because it only satisfies one inequality
0 is not a solution because it only satisfies one inequality
-1 is not a solution because it satisfies neither inequality
Solving Compound Inequalities Example #5:
Solve for x: x+2 < 0 and 8x+1 ≥ -7
Just as before, go ahead and solve each inequality as follows:
After solving both inequalities, we are left with x<-2 and x≥-1
Step #1: Identify if the solving compound inequalities problem is or or and
Note that his final example will demonstrate why step #1 is so important. Remember that solving this compound inequality requires you to find values that satisfy both x<-2 and x≥-1
Step #2: Graph both inequalities on the number line.
Again, this is an and problem, which means that you are looking for the intersection or overlap of the two lines on your compound inequality graph.
Step #3: Analyze and determine the solution set.
Notice anything strange about this example? There is actually no area where the inequalities intersect!
Since we are looking for values that satisfy both inequalities,
We can conclude that there are no solutions because there is no value for x that is both less than -2 and greater than or equal to -1.
Conclusion: How to Solve Compound Inequalities Using Compound Inequality Graphs in 3 Easy Steps
You can solve any compound inequality problem by apply the following three-step method:
Step #1: Identify if the solving compound inequalities problem is or or and
Solutions to or compound inequality problems only have to satisfy one of the inequalities, not both.
Solutions to and compound inequality problems must satisfy both of the inequalities.
It is possible for compound inequalities to zero solutions.
Step #2: Graph both inequalities on the number line.
Step #3: Analyze and determine the solution set.