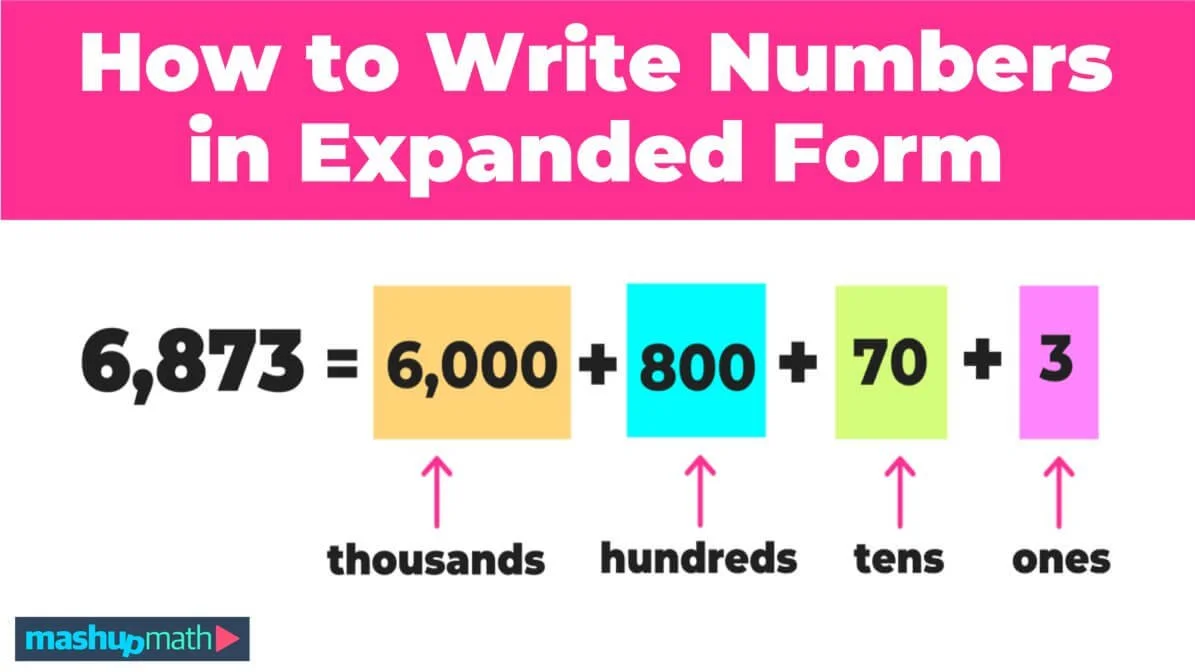
How to Find X and Y Intercepts

Finding the x and y intercepts of a graph means identifying the (x,y) coordinates where the graph intercepts the x-axis and the y-axis. You can find the x-intercept by setting y=0 and solving for x. And, you can find the y-intercept by setting x=0 and solving for y.

How to Find X and Y Intercepts

In math, the x-intercept and the y-intercept of a function are the coordinate points where the line or curve crosses the x-axis (the horizontal axis) and the y-axis (the vertical axis).

For example, by looking at the graph of the function y = x + 3, you can see that:

• The x-intercept is at (-3,0)

• The y-intercept is at (0,3)

When you are given the graph of a function, you can easily find the x and y intercepts by locating the coordinate points where the graph crosses the x-axis and the y-axis.

But, how do you find the x and y intercepts when you do not have a graph?

Being able find the x and y intercepts of a given function is a useful skill because it allows you to understand the behavior of the function, what it looks like, and how to sketch its graph on the coordinate plane.

How to Find X- and Y-Intercepts in 3 Steps

You can find the x- and y-intercepts of a function by following these three steps":

Finding the X-Intercept:

• Step 1: Substitute y=0 into the function.

• Step 2: Solve for x.

• Step 3: Express the result as a coordinate point in the form (x,0)

Finding the Y-Intercept:

• Step 1: Substitute x=0 into the function.

• Step 2: Solve for y.

• Step 3: Express the result as a coordinate point in the form (0,y)

For example, if you wanted to find the x- and y-intercepts of the function y = x + 3 without a graph, you could follow the three-step process as follows:

Finding the X-Intercept of y = x + 3

• Step 1: Substitute y=0 into the function

• y = x + 3 → 0 = x + 3

• Step 2: Solve for x.

• 0 = x + 3

• -3 = x → x = -3

• Step 3: Express the result as a coordinate point in the form (x,0)

• The x-intercept is (-3,0)

Finding the Y-Intercept of y = x + 3

• Step 1: Substitute x=0 into the function.

• y = x + 3 → y = 0 + 3

• Step 2: Solve for y.

• y = 0 + 3

• y = 3

• Step 3: Express the result as a coordinate point in the form (0,y)

• The y-intercept is (0,3)

Final Answer: The function y=x+3 has an x-intercept at (-3,0) and a y-intercept at (0,3).

This final answer should make sense since we already knew the x- and y-intercepts of this function by looking at the graph in Figure 01 above. Now, let’s get some more practice with finding x and y-intercepts by working through some step-by-step practice problems.

Example #1: Find the X and Y Intercepts

Problem: Find the x-intercept and the y-intercept of y = 2x + 1

We can find the x- and y-intercepts of the function y = 2x + 1 by following our three step process for each intercept as follows:

Finding the X-Intercept of y = 2x + 1

• Step 1: Substitute y=0 into the function

• y = 2x + 1 → 0 = 2x + 1

• Step 2: Solve for x.

• 0 = 2x + 1

• -1 = 2x → x = -1/2

• Step 3: Express the result as a coordinate point in the form (x, 0)

• The x-intercept is ( -1/2 , 0)

Finding the Y-Intercept of y = 2x + 1

• Step 1: Substitute x=0 into the function.

• y = 2x + 1 → y = 2(0) + 1

• Step 2: Solve for y.

• y = 2(0) + 1

• y = 0 + 1

• y = 1

• Step 3: Express the result as a coordinate point in the form (0,y)

• The y-intercept is (0,1)

Final Answer: The function y=2x+1 has an x-intercept at (-1/2,0) and a y-intercept at (0,1).

Example #2: Find the X and Y Intercepts

Problem: Find the x-intercept and the y-intercept of y = (x-2)/2

You can find the x- and y-intercepts of y = (x-2)/2 as follows:

Find the X-Intercept of y = (x-2)/2

• Step 1: Substitute y=0 into the function

• y = (x-2)/2 → 0 = (x-2)/2

• Step 2: Solve for x.

• 0 = (x-2)/2

• 0 = x - 2

• 2 = x

• Step 3: Express the result as a coordinate point in the form (x, 0)

• The x-intercept is ( 2 , 0 )

Finding the Y-Intercept of y = (x-2)/2

• Step 1: Substitute x=0 into the function.

• y = (x-2)/2 → y = (0-2)/2

• Step 2: Solve for y.

• y = (0-2)/2

• y = -2/2

• y = -1

• Step 3: Express the result as a coordinate point in the form (0,y)

• The y-intercept is (0,-1)

Final Answer: The function y = (x-2)/2 has an x-intercept at (2,0) and a y-intercept at (0,-1).

Example #3: Find the X and Y Intercepts

Problem: Find the x-intercept and the y-intercept of y = x² - 4

In the previous examples, we had to find the x and y-intercepts of linear functions, which cross the x and y-axis only one time. In this next example, we will look at a quadratic function, which can cross the x or y-axis multiple times.

Find the X-Intercept of y = x² - 4

• Step 1: Substitute y=0 into the function

• y = x² - 4 → 0 = x² - 4

• Step 2: Solve for x.

• 0 = x² - 4

• 4 = x²

• √(4) = √(x²)

• ± 2 = x → x = ± 2 → x = 2 and x = -2

• Step 3: Express the result as a coordinate point in the form (x, 0)

• The function has two x-intercepts: ( 2 , 0 ) and ( -2 , 0 )

Finding the Y-Intercept of y = x² - 4

• Step 1: Substitute x=0 into the function.

• y = x² - 4 → y = (0)² - 4

• Step 2: Solve for y.

• y = (0)² - 4

• y = 0 - 4

• y = -4

• Step 3: Express the result as a coordinate point in the form (0,y)

• The y-intercept is (0,-4)

Final Answer: The function y = x² - 4 has x-intercepts at (2,0) and (-2,0) and a y-intercept at (0,-4).

Real-World Problem: Find the X and Y Intercepts

Problem: The path of a rocket can be modeled by the equation y = -x² +5x, where x is the horizontal distance traveled by the rocket (in meters) from the launch point, and y is the height (in meters) above the ground. What is the total horizontal distance travelled by the rocket (in meters)?

To solve this real-world problem, we have to find the total horizontal distance travelled by the rocket. We can do that by finding the coordinates of the x-intercepts as follows:

Find the X-Intercept of y = -x² +5x

• Step 1: Substitute y=0 into the function

• y = -x² +5x → 0 = -x² +5x

• Step 2: Solve for x.

• 0 = -x² +5x

• 0 = x(-x+5)

  Solve each factor for zero:

• x=0

• -x +5 = 0 → x=5

• Step 3: Express the result as a coordinate point in the form (x, 0)

• The function has two x-intercepts: ( 0 , 0 ) and ( 5 , 0 )

While we do not know what the exact graph of y = -x² +5x, we know that it is a curve with x-intercepts at (0,0) and (5,0).

Therefore, we can conclude that the rocket travelled horizontally 5 meters.

Final Answer: The total horizontal distance travelled by the rocket was 5 meters.

Finding X and Y-Intercepts Interactive Quiz

Are you ready to assess your understanding of how to find x and y-intercepts? Once you have completed the practice problems above, go ahead and try the free interactive quiz below and see if you can correctly answer all five questions.

Finding X and Y Intercepts FAQ

What are x and y intercepts?

In math, the x-intercept of a function is where the graph crosses the x-axis, and the y-intercept of a function is where the graph crosses the y-axis. X-intercepts are of the form (x,0) and y-intercepts are of the form (0,y).

How do you find x and y intercepts?

You can find the x-intercept(s) of a function by following these three steps:

• Step 1: Substitute y=0 into the original equation.

• Step 2: Solve for x

• Step 3: Write the coordinate point in the form (x,0)

You can find the y-intercept(s) of a function by following these three steps:

• Step 1: Substitute x=0 into the original equation.

• Step 2: Solve for y

• Step 3: Write the coordinate point in the form (0,y)

Note that functions can have more than one x-intercept or y-intercept.